You are now entering the officially wonderful and weird world of transformations and symmetry. It transpires that symmetry is not only observed on the macro level (our bodies are basically symmetric to look at), but on the quantum level as well. Symmetry plays a huge role in our understanding of how the universe functions.
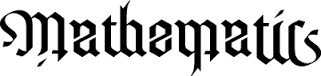The most basic forms of symmetry are reflective symmetry (or line symmetry) as shown in Scott Kim’s ambigram of the English alphabet at left, and rotational symmetry as shown in his design of the word mathematics at right (view it upside down).
I first encountered Scott Kim‘s work in OMNI magazine in the late 1970s. I would love to show you many examples of what he does with symmetry and agree with him that this artistic skill should be included in mathematics lessons. Please visit Scott Kim’s website and take time to read some of his blogs about mathematics and teaching (as well as seeing samples of his amazing artwork)!
Mathematicians are fascinated by symmetry and, ultimately, ask questions about symmetries that are not simply geometric. There are symmetries in algebra and relationships in quite a few areas of mathematics. They all have the idea of balance or of conserving something.
I hope you enjoy this journey with me.
I was tutored by Graeme for the majority of my high school years in mathematics. He really helped me to understand ways of working out problems that I had difficulty grasping, which I’m very thankful for! When I was studying some subjects in accountancy and business mathematics at university, I found the concepts that he had taught me were invaluable. He is a fantastic tutor, highly recommend him if you need any help with mathematics.
Jonathan T (student, c2005-2011)
See all Testimonials
