India
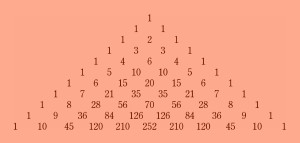
The triangle, and the means of constructing it (by adding the two terms above) were known by Pingala about two thousand years ago. He wrote the Chandaḥśāstra (also known as the Chandaḥsūtra), which was a study of metre in Sanskrit poetry. His discussion of light (laghu) and heavy (guru) syllables led him to a discussion of binary arithmetic. This corresponds to the binomial theorem upon which Pascal's Triangle is based.
Centuries later, the Indian astronomer and mathematician, Varāhamihira (505–587) also discussed the triangle and its additive properties. About 300 years later, about 850, the Jain mathematician Mahāvīra produced a means of generating the binomial coefficients by using multiplication. There are claims that his method is equivalent to the modern binomial formula which uses factorial notation. And, during the tenth century, another Indian mathematician, Halayudha, provides us with a description of this triangle which he calls Meru-prastaara (the Staircase of Mount Meru). It is through his commentary on Pingala's Chandaḥśāstra that we know of Pingala's use of Pascal's Triangle.
Persia (Iran)
The Persian mathematician, Al-Karaji (953–1029) wrote about Pascal's Triangle and the binomial theorem. A century later, the poet-astronomer-mathematician Omar Khayyám (1048–1131) also discussed Pascal's Triangle in his famous Treatise on Demonstration of Problems of Algebra (1070). Consequently, the triangle is referred to as the Khayyam triangle in Iran.
China
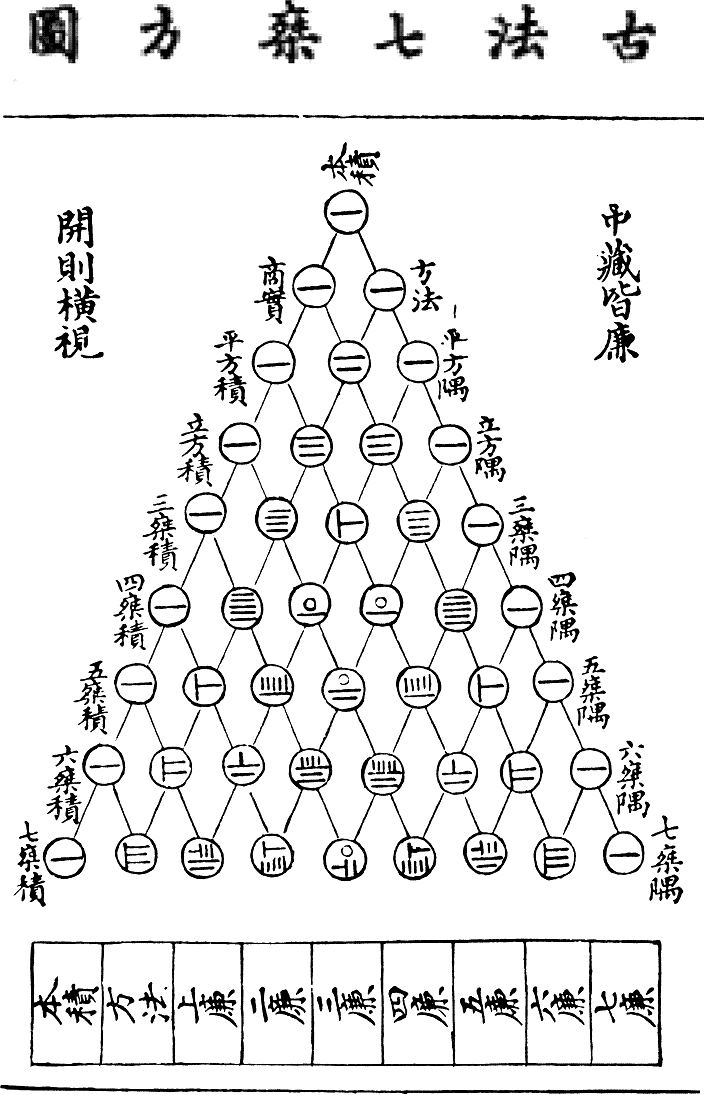 It is believed that the Chinese mathematician Jia Xian (1010–1070) invented Pascal's Triangle independently and he certainly used it as a tool for extracting square and cube roots.
It is believed that the Chinese mathematician Jia Xian (1010–1070) invented Pascal's Triangle independently and he certainly used it as a tool for extracting square and cube roots.
About 200 years later, Yang Hui (1238–1298) discussed Jia Xian's method (for finding roots using the triangle) at length and explicitly acknowledged Jia Xian as the source.
It is still called Yang Hui's Triangle (and occasionally, Jai Xian's Triangle) in China today. The illustration at right is from a publication by another Chinese mathematician, Zhu Shijie in his famous work, Jade Mirror of the Four Unknowns (1303).
Europe
The Jewish-French mathematician, astronomer and philosopher, Gersonides (Levi ben Gershon, 1288–1344) calculated the binomial coefficients using the multiplicative formula. [As an aside, he was also the first person to experimentally falsify the Ptolemaic model of the universe (by observing the brightness of Mars, among other things) and appears to have been the first to argue that the stars were billions of times further away than others had calculated.]
In 1527, the German mathematician, astronomer and cartographer, Petrus Apianus (1495–1552) published a copy of the triangle on the frontispiece of his handbook of commercial arithmetic, Ein newe und wolgegründete underweisung aller Kauffmanns Rechnung in dreyen Büchern, mit schönen Regeln und fragstücken begriffen. Not long afterwards, in his Arithmetica integra (1544), Michael Stifel (1487-1567), a German monk, published a portion of the triangle (from the second to the middle column in each row), describing it as a table of figurate numbers.
Only twelve years later (1556), the Italian algebraist Niccolò Fontana Tartaglia (1500–1577) published six rows of the triangle in General Trattato di numeri, et misure. In Italy, Pascal's triangle is referred to as Tartaglia's triangle.
In 1570, the Italian mathematician Gerolamo Cardano published the triangle and explained the additive and multiplicative rules for constructing it ...
... which brings us to Blaise Pascal and his work!
Around 1654, Blaise Pascal (1623-1662) was corresponding with his fellow French mathematician, Pierre de Fermat (1601-1665) concerning some matters relating to probability theory and its application to resolving some problems in gambling. Because of the triangle's usefulness in binomial theory and, therefore, in probability, he wrote a detailed analysis of it, Traité du triangle arithmétique (Treatise on the Arithmetic Triangle). It was published posthumously, in 1665. What made this work stand out from all preceding texts was that Pascal's was the first treatise in history that was completely devoted to analysing the triangle.
In 1708, Pierre Raymond de Montmort (1678-1719) called the triangle the "Table de M. Pascal pour les combinaisons" (Table of Mr Pascal for Combinations) and in 1730, Abraham de Moivre (1667-1754) referred to it as "Triangulum Arithmeticum Pascalianum" (Latin for "Pascal's Arithmetic Triangle").
Since those days it has been known as Pascal's Triangle in the West.